
Introduction to Schenkerian Analysis:
"The effect of being passing"
A Philosophical Basis for the Importance of Analysis
Dubiel (1990):
"Hearing [a piece] well means realizing how everything about it contributes in a variety of ways to a very full sense of how it is."
[Regarding analysis:] "'Rightness' is not an issue only richness of result is; which is incidentally to say that discourse between holders of contrasting attributive theories is not relevantly directed toward ranking and possible correction of the theories but toward exchange and exercise of them, to the end of giving both participants more options for how to listen."
"In short, the thought processes that constitute music have everything to do with figuring out not necessarily determining, but perhaps deciding how something might be heard, what it would take to hear it that way, what would happen if it were heard that way: quite a bit besides what the passage is."
Easy Navigation of this Document:
This document is designed to offer theoretical evidence for the importance and purpose of music analysis in the work of all musicians. Music analysis offers the most useful medium for the invention and exploration of musical interpretations. Performers who use music analysis effectively will find it a worthwhile method for finding the kind of musical richness they desire in their interpretations. In order to appreciate the points made here, one must have an understanding of Species Counterpoint and basic Schenkerian analysis. For a beginners' guide to Schenkerian analysis and a summary of the Schenkerian view of Species Counterpoint, see Tom Pankhurst's Schenker Guide. The "effect of being passing" is a concept of Schenkerian theory mentioned in Dubiel (1990) and Snarrenberg (1997) that will be developed here with the purpose of showing how analysis can offer musicians fuel for interpretive decision-making. Most ideas in this document are taken from one or more of the sources listed in the Bibliography. Rather than give a footnote nearly every sentence, I direct the reader's attention especially to Komar 1971 and Larson 1997 for part 1, Benjamin 1982, Cohn and Dempster 1992, and Dubiel 1990 for part two, and Benjamin 1981 and Jones 2002 for part three.
The process of composing-out (auskomponierung) will be described here as a function of adding diminutions, or ornamental figures, onto a stable harmony. The example below shows a tonic chord in C major. The chord is relatively stable because it is in root position, but it is not as stable as a tonic chord with scale degree 1 in the soprano.

An artist would most likely desire a more interesting and more conclusive basic background pattern on which to build his piece. The composer now uses a consonant skip to provide (the simplest kind of) harmonic prolongation and to end on a more stable tonic than the opening tonic. ("Prolongation" will be defined in increasing detail throughout the document.)

The melodic line that this consonant skip has created is not particularly "fluent." It follows "nature" in that it descends through the partials of the harmonic series of the tonic note, but it needs also to follow the nature of the human voice by exhibiting stepwise melodic motion. Therefore, the composer adds a dissonant passing tone between scale degree 3 and scale degree 1. The passing tone is not a member of the tonic chord, and is thus quite unstable. It simply serves to provide a path between two more stable notes, thus prolonging their function. The "effect of being passing" is thus the idea that an unstable transitory span between two more stable entities serves to enhance or extend their effect. Without the use of passing events to create prolongation, all music would fail to be ambiguous, metaphoric, or poetic.

To aid in the passing tone's ability to prolong tonic function over a long span of music, it is now harmonized using the dominant. According to Schenker, a dissonant note cannot be prolonged by further diminutions. To allow for further prolongation, the V chord now gives scale degree 2 consonant support. This does not destroy the "effect of being passing" between the two more stable tonic chords (see the quote below).

Because of its consonant support, scale degree 2 can now serve as a structural harmony on which more diminutions can be added. "A consonant chord brought into existence to harmonize a dissonant passing tone takes on the passing nature of the tone, and retains it even under subsequent transformations that change its voicing and produce more chords in elaboration of it; indeed "Schenker's theory" as we know it can be construed as an enormous extension [prolongation?] of this claim. Schenker can use the passing tone as the basis for his entire model of musical coherence precisely because he believes that the effect of "being passing" can never be drowned out.... When several lines present passing tones together, forming consonant intervals between them, Schenker will speak directly of "dissonant effects by means of detours through consonant sounds" (II, 208)." (Dubiel 1990) These diminutions, as well as passing events added to any other part of the structure, can be harmonized, and themselves be prolonged in a recursive cycle (creating many hierarchical layers of music, or Schichten), in infinitely many different ways, so as to produce almost any piece of tonal music. This background pattern created by harmonizing scale degree 2 can thus be used as an Ursatz, or basic structure for all tonal music (as shown below). "Ultimately the Ursatz, whatever else it may be, is an assertion that most of the events of a piece, however protracted, stable, and locally well defined and independent, are passing toward the final tonic." (Dubiel 1990)
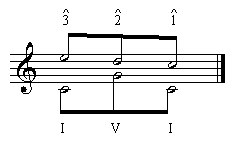
In Schenker's view, any type of diminution or prolongation (CS, P, N, etc.) has the effect of being "passing" between contextually stable sonorities. Any diminutions added to the music at any level should ideally follow the laws of species counterpoint, or at least acknowledge the effects of breaking certain rules. The structural tones at any hierarchical level of the music will therefore serve as a cantus firmus upon which the composer improvises, adding ornamentation according to good contrapuntal practice, the desired musical effect, and the creation of motivic parallelisms among musical layers (Schichten). Thus, the word "prolongation" serves more than one function for Schenker: The extension of the length of a musical structure through the use of diminutions is the first and most literal use of the word. But also, according to Dubiel (1990), "Prolongation ... means no more than Schenker's compulsively repeated slogan Semper idem sed non eodem modo. It amounts to an insistence that apparent departures from the [species counterpoint] rules be understood somehow as extensions of them." The great composers thus know the effects of the contrapuntal procedures, and compose according to the necessity of creating certain effects, "prolonging" the rules and the musical structure as required. (At this point, rereading the first section of this document with this expanded understanding of the word "prolongation" in mind may enrich your understanding of the "effect of being passing".)
The Importance of Hearing from Background to Foreground
In light of the fact that the passing tone forms the cornerstone of Schenker's view of music, one can now see why the Schenkerian reading of a piece from the background to the foreground must be audible to the analyst. The contextual stability of the tonic areas that frame a tonal work, and the relative instability of the prolonging intermediate events that create the sense of tonal motion and development, are essential to the expression of functional tonality. The "effect of being passing" must consequently enter into the musical interpretation of any piece, if that piece is to make sense as tonal music. This is not to diminish any other view of a tonal piece, whether motivic, formal, contrapuntal, melodic, harmonic, or semiotic. Schenkerian analysis allows the interpreter to explore the tonal aspects of a piece, and offers a flexible structure on which to hang the results of all other analytical methods. "Richness of result" is the goal, not "rightness", or rigidness, or rejection of any analytical method because of contradictory results.
Functions: "Things for things to be"
In any piece, Schenkerian (and other forms of) analysis should reveal compositional purposes, musical and extramusical associations, and ideas to bring into one's listening experience of the piece. "Functions" are thus the effects of following or breaking rules mentioned earlier in connection with contrapuntal practice. In this light it is important to assert that a Schenkerian view of hierarchical levels is that the higher, more abstract levels of an analysis bear a metaphoric relationship with the musical surface. That is, they are a way of understanding the surface as a complex combination (prolongation) of simpler musical ideas representable by different middleground graphs according to the analysts' musical intuitions and insights. Dubiel (1990) calls these functions "things for things to be" or more specifically effects, ideas, and Schenkerian readings to try out and see "what it would take to hear it that way, [and] what would happen if it were heard that way." It is therefore important for analysts to try out multiple readings of a piece, and as long as the readings offer rewarding insights into the compositional effect work on incorporating the richness provided by each into a sort of meta-reading of the surface.
Functions can thus be any aspect of a Schenkerian graph including the "effect of being passing", or more simple functions such as Riemannian chord function, or a passage's function as a development of the main motives of the piece, or the extramusical associations of a passage, or any other kind of musical insights that come from thorough analysis. It is always important for a listener to be making associations between structures in the music and structures in the mind: in one's knowledge of musical patterns, rudiments, and fundamental structures; in one's memory of different musical ideas and patterns in this and other musical works; and in one's understanding of extramusical ideas, structures, and metaphors.
The richness of the analysis will be exhibited in the performance of the work by a musician who has such a full understanding of the effects and functions. A performer who understands and can hear the relationship between a recurring melodic fragment and an underlying hidden parallelism will arguably play the music differently and more communicatively than one who simply has so-called "musical intuition" about the way the music should be interpreted. Analysis is thus a tool for bringing interpretive insights into focus and allowing them to be communicated either verbally, or aurally in performance.
Pitch-Class Counterpoint and the Diatonic Lattice
Traditional Schenkerian analysis deals largely with counterpoint, treating the dissonances and diminutions of species counterpoint as surface decorations of more basic first-species two-voice structures. A contrapuntal theory of prolongation and structural levels nevertheless also functions effectively with a harmonic underpinning for composing-out. (Agmon 1996 suggests a theory of functional prolongation that resonates with this theory.) Any non-chord tones and structurally subordinate harmonies will exhibit recognizable contrapuntal patterns with "passing" status relative to the structural harmonies. These may not always appear in literal pitch structures, but rather will be found in the contrapuntal activity expressed by the pitch-classes underpinning a progression. As Schenkerian analysis takes a fundamentally diatonic perspective, this contrapuntal activity is best expressed in mod-7 space. Jones (2002) has devised a "diatonic lattice" structure for graphing the pitch-class counterpoint and observing passing and neighboring motions among pitch-classes participating in the chord progressions of any piece. For more information on the diatonic lattice and expressing harmonic progressions on the mod-7 framework, see Prolongation and the Diatonic Lattice.
"The effect of being passing" Formalized
Any prolongation that would be part of a Schenkerian graph of a piece will also be expressible as contrapuntal motion on the diatonic lattice. Jones (2002) has formalized the criteria for observing the "effect of being passing" on the lattice. According to Jones, any prolongation between two harmonies exhibits passing and neighboring motions in the pitch-class counterpoint between all chord members of both the initiating and terminating chords. Jones defines passing motion as stepwise ascent or descent that may linger on a pitch class along the way but never changes direction. Neighbor motion is defined as a single stepwise alternation figure with only the next higher or lower diatonic step, holding for as long as necessary on each of these two steps, but only leaving from and returning to the original scale step once. When every member of the first chord passes in one of these manners to some member of the last chord, and every member of the last chord concludes a passing or neighboring line from some member of the first chord, the progression is called "pervasively fluent" (after Schenker's "melodic fluency"). The diatonic pitch-class lines contributing to a pervasively fluent passage (or PFP) provide the means by which a passage can be heard as being harmonically transient (or "being passing") between two structural chords.
"What it would take to hear it that way"
It is important to realize that the requirements for a progression to be a PFP are such that all three-chord progressions of three- and four-voice chords are by definition pervasively fluent. Certainly some passages that can be analyzed as being pervasively fluent would not offer particularly worthwhile insights into the music when they are analyzed as prolongations. It is in the nature of the considerable abstraction of pitch-class counterpoint, though, that the lattice structures can be imagined as being many "things" (analytic functions) that each offer either less or more richness to the resulting interpretation. It is therefore the responsibility of the analyst a priori to choose analytically rewarding prolongations based on Schenkerian theory for exploration as PFPs. The PFPs merely offer the passing and neighboring lines to try to hear in one's listening of the piece. Analysis of a passage on the diatonic lattice as a potential prolongation is thus a technique for discovering and exploring "what it would take to hear it that way."
Exploration of harmonic prolongations as PFPs can be accomplished with the PFP Finder Applet. For an introduction to the use of the applet, see Prolongation and the Diatonic Lattice.
For more complete bibliographies, see:
Callon, Gordon J. [Online] Theory & Analysis Resources
Pankhurst, Tom. http://www.schenkerguide.com/bibliography.html.
Schenkerian Analysis Textbooks:
Cadwaller, Allen and David Gagné. 1998. Analysis of Tonal Music: A Schenkerian Approach. New York: Oxford University Press.
Forte, Allen and Steven E. Gilbert. 1982. Introduction to Schenkerian Analysis. New York: W.W. Norton.
"The effect of being passing":
Agmon, Eytan. 1996. "Conventional Harmonic Wisdom and the Scope of Schenkerian Theory: A Reply to John Rothgeb (MTO 2.1)." Music Theory Online 2/3. <http://societymusictheory.org/mto/issues/mto.96.2.3/mto.96.2.3.agmon.html>.
Benjamin, William E. 1981. "Pitch-Class Counterpoint in Tonal Music." In Richmond Browne, ed., Music Theory: Special Topics (New York: Academic Press), 1-32.
. 1982. "Models of Underlying Tonal Structure: How Can They Be Abstract, and How Should They Be Abstract?" Music Theory Spectrum 4: 28-50.
Cohn, Richard, and Douglas Dempster. 1992. "Hierarchichal Unity, Plural Unities: Toward a Reconciliation." In Katherine Bergeron and Philip Bohlman, eds., Disciplining Music: Musicology and Its Canons (Chicago: University of Chicago Press), 156-181.
Dubiel, Joseph. 1990. "'When You Are A Beethoven': Kinds of Rules in Schenker's Counterpoint," Journal of Music Theory 34/2: 291-340.
Jones, Evan. 2002. "Pervasive Fluency: A Contrapuntal Definition of Stability and Transience in Tonal Music." Ph.D. diss., University of Rochester.
Komar, Arthur J. 1971. Theory of Suspensions: A Study of Metrical and Pitch Relations in Tonal Music. Princeton, N.J.: Princeton University Press.
Larson, Steve. 1997. "The Problem of Prolongation in Tonal Music: Terminology, Perception, and Expressive Meaning." Journal of Music Theory 41/1: 101-136.
Snarrenberg, Robert. 1997. Schenker's Interpretive Practice. New York: Cambridge University Press.
Wagner, Naphtali. 1995. "No Crossing Branches? The Overlapping Technique in Schenkerian Analysis." Theory and Practice 20: 149-175.
Return to Pedagogical Resources
Return to PFP finder
Return to Robert Kelley's Music Website
© 2002 . All rights reserved. This work is the intellectual property of Robert T. Kelley. Reproduction of any part of or ideas from this document without is unlawful.